The lecture takes place in the summer semester.
Lecturer: Apl. Prof. Dr. Sc. Tatiana Gambaryan-Roisman
Introduction
In this course you will learn a selection of analytical/mathematical methods for solving heat transfer problems that can be described by partial differential equations. Applications include heat conduction, convective heat transfer, and phase changes such as evaporation or solidification.
The learning objective is to provide you with a powerful and comprehensive toolbox of methods to successfully deal with the complex problems of heat transfer and other transport phenomena, also in combination with the numerical methods and experiments.
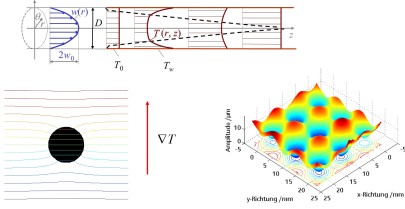
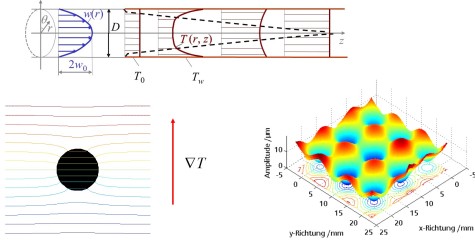
Course content
- Introduction
- Differential equations in heat transfer: Definitions and examples
- Methods of solution of linear partial differential equations
- Separation of variables
- Fourier series
- Sturm – Liouville problems
- Special functions
- Integral transforms
- Introduction into nonlinear problems and advanced solution methods
- Perturbation methods
- Heat transfer in thin films
- Stability analysis
Literature
A summary is distributed weekly in the lectures.
Prerequisites
basic knowledge in mathematics and heat transfer
Online services
Moodle: lecture slides, videos, Matlab-Skripts
Further information: Course announcement summer semester 2025 (opens in new tab)

